Advertisement
If you have a new account but are having problems posting or verifying your account, please email us on hello@boards.ie for help. Thanks :)
Hello all! Please ensure that you are posting a new thread or question in the appropriate forum. The Feedback forum is overwhelmed with questions that are having to be moved elsewhere. If you need help to verify your account contact hello@boards.ie
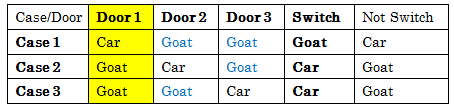
3 doors - One contains a brand new car, the other two contain a goat. Choose.
-
24-09-2017 08:34PM#1
Comments
-
-
-
-
-
-
Advertisement
-
-
-
-
-
-
Advertisement
-
-
-
-
-
-
-
-
-
-
-
Advertisement
-
-
-
-
-
-
-
-
-
-
Advertisement
-
Advertisement




 https://www.youtube.com/watch?v=yuwprXAaSv0
https://www.youtube.com/watch?v=yuwprXAaSv0